
Variance of coin flip pdf#
I did, however, find a PDF of a book written in 1940 by the very people that the runs test is named after: “On a test whether two samples are from the same population”, by Wald and Wolfowitz. Every source I found cited textbooks that I do not have easy access to (this should be remedied once I’m an official Masters student this fall). The expectation formula was simple to solve in a matter of minutes, but for some reason, I couldn’t find a proof of the variance anywhere on the internet (after an hour of searching).

I was on a mission to find the expectation and variance for this problem. We use the word “runs” to denote consecutive outcomes of the same result. By calculating the expectation (mean) and variance of the number of runs in this type of experiment, we can use the normal distribution to approximate the probability that we get a certain number of runs. The runs test can analyze both experiments to see which one is more likely to be a truly random outcome of flips. Intuitively, if someone flips a coin 10 times and gets 5 heads and 5 tails, it is more plausible that the order of outcomes H, T, T, H, T, H, H, H, T, T is more likely to be random than something like H, H, H, H, H, T, T, T, T, T. More precisely, it can be used to test the hypothesis that the elements of the sequence are mutually independent.” In layman’s terms, it can be used to test the randomness of a distribution comprised of only two possible outcomes (heads and tails for example). According to Wikipedia, this is a “statistical test that checks a randomness hypothesis for a two-valued data sequence. There’s actually a straightforward approach to solving this problem that involves the geometric distribution, but after researching ways to approach this problem, I stumbled across something known as the Wald–Wolfowitz runs test (or simply runs test). HTTTHHTHTTHHTHHHTHTTHTTHTTTHHTHTHTTTTHTHTHTTHTHTHT HHHTTTTHTHHHTHHTHTHTHTHHHTHTTHHHTHHHHHHTHHHTHTTTHH THTHHTTHTTHTHTHTTHHHHHTTHTTTHTHTHHTTTTHTTTTTHHTHTH TTTTHTHTTHHHHTHHHHHHHHTTHHTHHTHHHHHHHTTHTHTTTHHTTT THTTTTHHHHTHTHTTHTTTHTTHTTTTHTHHTHHHHTTTTTHHHHTHHH HTHHHTHTTHHTTTTTTTTHHHTTTHHTTTTHHTTHHHTTHTHTTTTTTH HHTHTHTHTHHHTTHHTHTTTHTHHTHTHTHHTHTTHTTHTHHTHTHTTT Sequence 2: HTHTHTHHHTHTHTHTHHTHHTHTHTTHTTTHHTHTTTHTHHTHHHHTTT THHTTHTHTTHHHTHTHTHTTHTTHHTTHTHHTHHHTTHHTHTTHTHTHT TTHTHTHTHTHTTHTHTHHHTTHTHTHHTHHHTHTHTTHTTHHTHTHTHT TTHTHHTHTTTHHTTHHTTHHHTHHTHTTHTHTTHHTHHHTTHTHTTTHH TTHHTHTTHTTTHTTTHTTTHTTHTHHTHHTHTHHTTTHHTHTHTTHTHH Both sequences have 148 heads, two less than the expected number for a 0.5 probability of heads. Which sequence is the true sequence of coin flips? Make an argument that is justified with probabilities calculated on the sequences. The other was generated by a person typing out H’s and T’s and trying to seem random. One of these is a true sequence of 300 independent flips of a fair coin. The problem is as follows:īelow are two sequences of 300 “coin flips” (H for heads, T for tails).
Variance of coin flip how to#
A few weeks ago, I had an idea for a homework problem but I couldn’t quite figure out how to implement the idea.
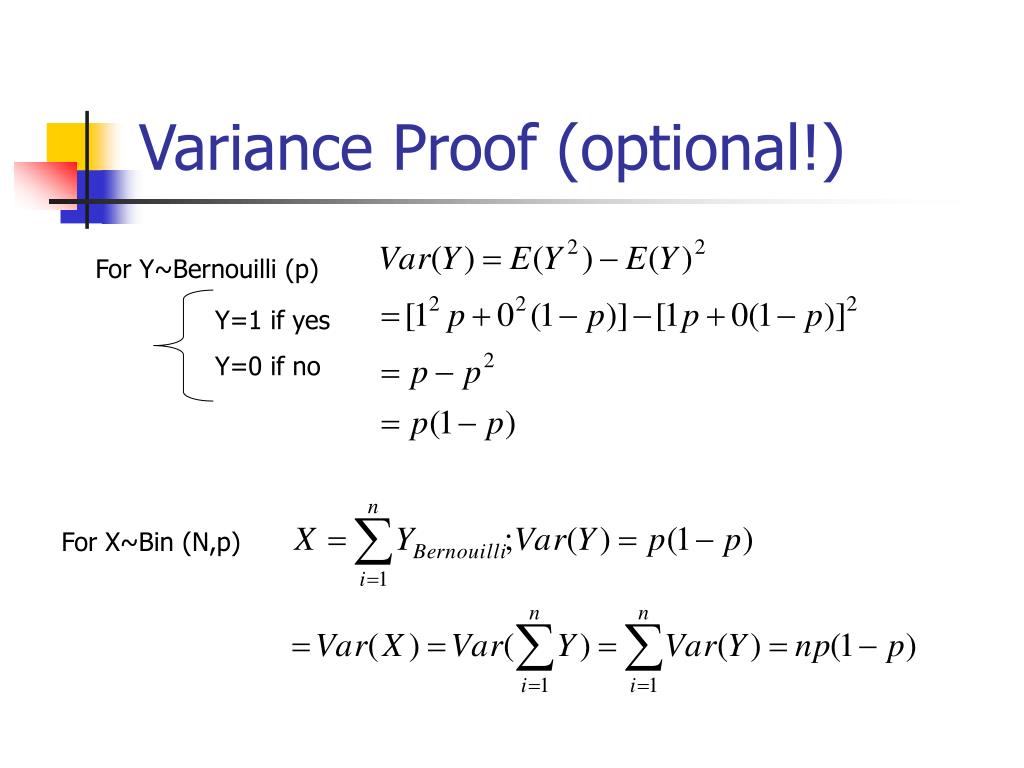
This 8-week long class was intense and challenging but one of the most rewarding classes I’ve taken at Stanford so far. Last week, I completed a summer course at Stanford: CS109, Probability for Computer Scientists. Note: knowing intermediate probability and calculus is necessary to understand this post.


 0 kommentar(er)
0 kommentar(er)
